Recall how we used the Hess’s law to calculate the enthalpy change (ΔH) for a reaction without having the enthalpies of formation for any or some of the components in the reaction.

On the basis of the Hess’s law is the fact that enthalpy is a state function, and therefore, it does not matter what route the conversion the molecules take, but rather what the enthalpies of the reactants and products are.

 

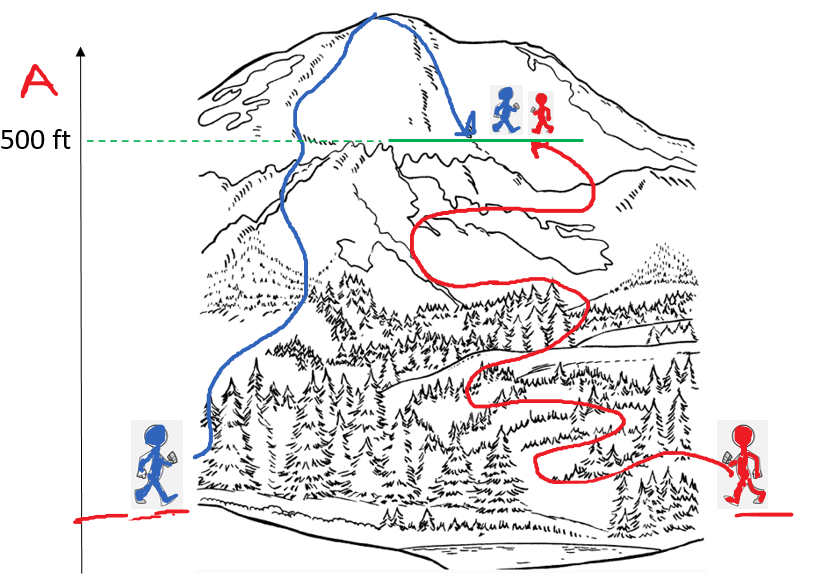
Remember, a state function is a variable that only depends on the initial and final states and now how it was achieved. For example, altitude is a state function and wouldn’t matter how two hikers meet at a 500 ft altitude. One may be on his way to the top, and the other going back having behind a longer walk distance:

 

 

Compare this to the enthalpy change for the following stepwise reaction converting molecules A and B to molecule C:

 

 

The enthalpy change is the same if molecules A and B are converted to C, and then D compared to if they go directly to D. Therefore, if we add the values of the enthalpies with their signs, the final result will be the enthalpy change of the net conversion.

Now, because Gibss free energy is also a state function, we can apply the same strategy and calculate the ΔG^o for a stepwise reaction from the sum of the changes in ΔG^o for each step.

 

For example, given the data for the three combustion reactions below, calculate the free energy of the reaction producing methanol (CH₃OH) from carbon monoxide and hydrogen gas.

 

CO(g) + 2H₂(g) → CH₃OH(g), ΔG^o = ?

1) 2CO(g) + O₂(g) → 2CO₂(g), ΔG^o= -514 kJ

2) 2H₂(g) + O₂(g) → 2H₂O(g), ΔG^o = -458 kJ

3) 2CH₃OH(g) + 3O₂(g) → 2CO₂(g) + 4H₂O(g), ΔG^o = −1378 kJ

 

Solution: The first two equations seem to be in the correct directions since they contain CO and H₂ as reactants which is what we have in the target equation.

The third equation, however, must be reversed because the methanol here is a reactant while in the target equation, it is a product. So, let’s reverse it and label as 3a:

 

3a) 2CO₂(g) + 4H₂O(g) → 2CH₃OH(g) + 3O₂(g), ΔG^o = +1378 kJ

 

Let’s add equations 1, 2, and 3a and see what we get:

 

1) 2CO(g) + O₂(g) → 2CO₂(g), ΔG^o = -514 kJ

+

2) 2H₂(g) + O₂(g) → 2H₂O(g), ΔG^o = -458 kJ

+

3a) 2CO₂(g) + 4H₂O(g) → 2CH₃OH(g) + 3O₂(g), ΔG^o = +1378 kJ

 

You may notice that there are four moles of water on the left side (equation 3a), but only two moles on the right side (equation 2). This means we need to multiply equation two with its ΔG value by two. Let’s do that and label the new equation as 2a:

 

2a) 4H₂(g) + 2O₂(g) → 4H₂O(g), ΔG^o = -916 kJ

 

And now, we can add equations 1, 2a, and 3a:

 

1) 2CO(g) + O₂(g) → 2CO₂(g), ΔG^o = -514 kJ

+

2a) 4H₂(g) + 2O₂(g) → 4H₂O(g), ΔG^o = -916 kJ

+

3a) 2CO₂(g) + 4H₂O(g) → 2CH₃OH(g) + 3O₂(g), ΔG^o = +1378 kJ

 

2CO(g) + O₂(g) + 4H₂(g) + 2O₂(g) + 2CO₂(g) + 4H₂O(g) → 2CO₂(g) + 4H₂O(g) + 2CH₃OH(g) + 3O₂(g)

 

Cancel the same molecules on both sides of the equation:

2CO(g) + O₂(g) + 4H₂(g) + 2O₂(g) + 2CO₂(g) + 4H₂O(g) → 2CO₂(g) + 4H₂O(g) + 2CH₃OH(g) + 3O₂(g)

ΔG^o = -514 kJ + (-916 kJ) + 1378 kJ/mol = -52 kJ

2CO(g) + 4H₂(g) → 2CH₃OH(g), ΔG^o = -52 kJ

 

The last step is to divide the equation by two to match the target reaction:

 

CO(g) + 2H₂(g) → CH₃OH(g), ΔG^o = -26 kJ

 

These types of problems are no different than what we did when discussing Hess’s law. Check the article and practice examples at the links.

 

Check Also

• Standard Entropy Change (𝚫S^o_rxn) of a Reaction
• The Gibbs Free Energy
• The Effect of 𝚫H, 𝚫S, and T on 𝚫G – Spontaneity
• Entropy and State Change
• Entropy Changes in the Surroundings
• 𝚫G^o_rxn from the Free Energies of Formation
• Gibbs Free Energy Under Nonstandard Conditions
• Gibbs Free Energy and Equilibrium Constant
• Entropy, Enthalpy, and Gibbs Free Energy Practice Problems