In the previous post, we talked about the Gibbs free energy for reactions at nonstandard-state conditions, ΔG. It is related to the free energy change of the reaction at standard-state conditions, ΔG^o, and the reaction quotient, Q with the following equation:

 

 

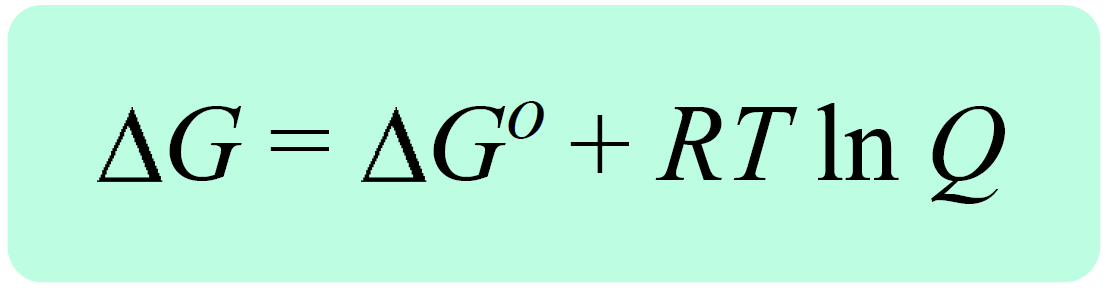
Remember, the reaction quotient is used to predict the direction of the equilibrium depending on the initial quantities of the reactants and products:

 

• Q < K Reaction tends to form more products.
• Q > K Reaction tends to form more reactants.
• Q = K Reaction is already at equilibrium.

 

Because the system always tries to reach an equilibrium, Q tends to become equal to K, and when that happens, an equilibrium is established, K = Q.

Now, by definition, when the equilibrium is established, the free energy of the reaction becomes a zero, ΔG = 0. So, if we replace Q with K, and ΔG with 0, we obtain a very important equation in chemistry that correlates the equilibrium constant the ΔG^o:

 

0 = ΔG° + RT ln K, therefore,

 

 

We can apply this equation to reactions in solutions, K_c and the ones involving gases in which case, K_P is used. The equation can also be used for the solubility constant, K_sp.

 

How do we interpret this equation?

The key link here is the negative sign which tells us the larger the K, the more negative ΔG° is. Remember, a larger K indicates that the products are favored which is essentially to say that the reaction is spontaneous – it tends to proceed forward producing more products. A smaller equilibrium constant, on the other hand, makes the ΔG° larger and if it becomes a positive value, the reaction is not spontaneous anymore. This happens when K < 1, because the RT ln K term becomes negative, and the minus sign makes the ΔG° positive. Again, K < 1 means that the equilibrium mixture is mainly reactants, and therefore, the forward reaction is nonspontaneous.

To summarize the correlation between the equilibrium constant the ΔG°, we can write that:

♦ When K < 1, ln K is negative and ΔG^o_rxn > 0. The reaction is not spontaneous.
♦ When K > 1, ln K is negative, and ΔG^o_rxn < 0. The reaction is spontaneous.
♦ When K = 1, ln K = 0, ΔG^o_rxn = 0. The reaction is at equilibrium.

 

The significance of the equation ΔG° = -RT ln K is evident when, for example, the equilibrium constant of the reaction cannot be determined experimentally. What we do then is calculate the ΔG° and use it to determine the equilibrium constant.

 

For example, using the data from an appendix for thermodynamic data, calculate the equilibrium constants of the following reaction at 25 °C:

 

NO(g) + O₃(g) ⇆ NO₂(g) + O₂(g)

 

Solution: The first is to determine the ΔG^o from the standard free energies of formation using the following equation:

ΔG°_rxn = Σn_pΔG^o_f (products) – Σn_rΔG^o_f (reactants)

 

NO(g) + O₃(g) ⇆ NO₂(g) + O₂(g)

ΔG° = [ΔG^o_f (NO₂(g)) + ΔG^o_f (O₂(g))] – [ΔG^o_f (NO(g)) + ΔG^o_f (O₃(g))]

ΔG° = [(52 + 0) – (87 + 163)] = -198 kJ

 

ΔG° = –RT ln K

                                                                                         \[\ln \,K\; = \;\frac{{ – \Delta G^\circ }}{{RT}}\]

 

Taking antilog to get an expression for K:

 

\[K\; = \;{e^{\frac{{ – \Delta G^\circ }}{{RT}}}}\]

 

You can either calculate the exponent term separately or use the numbers in this equation.

 

\[K\; = \;{e^{\frac{{{\rm{ – }}\left( {{\rm{ – 198}}\,{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{\rm{3}}}\,{\rm{J}}} \right)}}{{{\rm{8}}{\rm{.314}}\,{\rm{J/K}} \cdot {\rm{mol}}\, \cdot {\rm{298}}\;{\rm{K}}}}}}\, = \,{e^{79.9}}\; = \;5.01\, \times \,{10^{34}}\,\]

 

The equilibrium constant is very large, and this is in agreement with a highly negative ΔG°.

 

Check Also

• Standard Entropy Change (𝚫S^o_rxn) of a Reaction
• The Gibbs Free Energy
• The Effect of 𝚫H, 𝚫S, and T on 𝚫G – Spontaneity
• Entropy and State Change
• Entropy Changes in the Surroundings
• 𝚫G^o_rxn from the Free Energies of Formation
• Gibbs Free Energy and Hess’s Law
• Gibbs Free Energy Under Nonstandard Conditions
• Entropy, Enthalpy, and Gibbs Free Energy Practice Problems