In the previous post, we learned how to determine whether the solution of a salt is going to be acidic or basic depending on how it was prepared.

In short, there is only one step to do this and that is finding the strong component of the salt. If the salt is made of strong acid and a weak base, then it will give an acidic solution. If it is prepared from a strong base and weak aid, the solution will be basic, and if it is a combination of a strong acid and a strong base, a neutral solution will be formed.

 

 

Today, we will discuss calculating the pH of a salt solution.

 

The pH of Salt Prepared from a Strong Acid and a Weak Base

For example, calculate the pH of 0.10 M solution of NH₄Cl. K_b of NH₃ is 1.76 x 10^-5

Ammonium chloride (NH₄Cl) is the salt of ammonia (NH₃) and hydrochloric acid (HCl) and it yields an acidic solution. So, let’s follow what we have discussed earlier and see why it produces an acidic solution.

Step 1. Determine the acid and base that the salt is prepared from:

 

NH₃ + HCl → NH₄Cl

 

It is made of a weak base ammonia and a strong base HCl, and therefore, the solution is going to be acidic.

 

Step 2. Dissociate the salt and write the reaction of the weak component ion with water.

 

NH₄Cl → NH₄⁺ + Cl^–

 

Step 3. Write the reaction with water.

NH₄⁺ is the ion with a weaker component (NH₃), so it is going to react with water producing hydronium ion which makes the solution acidic:

NH₄⁺(aq)  + H₂O(l) → NH₃(aq)  +  H₃O⁺(aq)  (acidic)

 

The next part is to determine the concentration of H₃O⁺ ions in order to calculate the pH.

Step 4. Determine the K_a.

We are given the K_b, which measures the base strength of it and is based on the reaction of ammonia itself with water:

 

NH₃(aq) + H₂O(aq) ⇆ NH₄⁺(aq) + OH^–(aq)

 

This equation does not contain H⁺/H₃O⁺ ions, and we do not know the concentration of ammonia, therefore, we need to determine the K_a. K_a and K_b can be calculated from one another using the relationship:

 

K_a · K_b = K_w = 10^-14

 

Rearranging this, we get an expression for K_a:

 

\[{K_{\rm{a}}}\; = \,\frac{{{K_{\rm{w}}}}}{{{K_{\rm{b}}}}}\; = \;\frac{{{\rm{1}}{{\rm{0}}^{{\rm{ – 14}}}}}}{{{\rm{1}}{\rm{.76}}\; \times \;{\rm{1}}{{\rm{0}}^{{\rm{ – 5}}}}}}\; = \;{\rm{5}}{\rm{.7 }} \times {\rm{ 1}}{{\rm{0}}^{{\rm{ – 10}}}}\]

 

 Step 5. Set up an ICE table to Determine the [H₃O⁺]

Now that we know the value of K_a, we can set up an ICE table for K_a assigning x mol/l for ionization. From now on, it is the same procedure as we do when calculating the pH of a weak acid.

 

 

Next, write the expression for Ka using the equilibrium concentrations in the ICE table:

 

\[{K_{{\rm{a}}}}\; = \,\frac{{{\rm{[N}}{{\rm{H}}_{\rm{3}}}{\rm{][}}{{\rm{H}}_{\rm{3}}}{{\rm{O}}^{\rm{ + }}}{\rm{]}}}}{{{\rm{[N}}{{\rm{H}}_{\rm{4}}}^{\rm{ + }}{\rm{]}}}}\; = \;\frac{{{{\rm{x}}^{\rm{2}}}}}{{{\rm{0}}{\rm{.10}}\;{\rm{ – }}\;{\rm{x}}}}\; \approx \;\frac{{{{\rm{x}}^{\rm{2}}}}}{{{\rm{0}}{\rm{.10}}}} = \;{\rm{5}}{\rm{.7 x 1}}{{\rm{0}}^{{\rm{ – 10}}}}\]

 

Notice that we used an approximation that x is very small compared to the initial concentration of the salt. To check if the approximation was valid, calculate the precent ratio of x and the initial concentration:

                                                                   

\[\% \, = \;\frac{{7.5\, \times \;{{10}^{ – 6}}}}{{0.10}}\; \times \;100\% \; = \;0.0075\% \]

 

The hydrolyzation reaction percent is less than 5%, and therefore, the approximation is valid.

The concentration of H₃O⁺, so the pH is:

 

pH = -log 7.5 x 10^-6 = 5.1

 

This indicates an acidic solution which is what we expected to have for the solution of NH₄Cl.

  

The pH of Salt Prepared from a Strong Base and a Weak Acid

 For example, calculate the pH of 0.20 M solution of KCN. Ka for HCN is 4.9 x 10^-10

 Step 1. Determine the acid and base that the salt is prepared from.

Potassium cyanide (KCN) is prepared from a strong base (KOH) and weak acid (HCN), and therefore, its aqueous solution will be basic.

 

KOH + HCN → KCN + H₂O

 

Step 2. Dissociate the salt

 

KCN(aq) → K⁺(aq)  + CN^–(aq)

 

Step 3. Write the reaction of the weak component with water.

K⁺ comes from a strong base KOH, so does not react with water. CN^– comes from a weak acid HCN, so it will react with water.

 

CN^–(aq)  + H₂O(l) → HCN(aq)  +  OH^–(aq) (basic)

 

Step 4. We are given the K_a, so we need to determine the K_b.

 

\[{K_{\rm{b}}}\; = \,\frac{{{K_{\rm{w}}}}}{{{K_{\rm{a}}}}}\; = \;\frac{{{\rm{1}}{{\rm{0}}^{{\rm{ – 14}}}}}}{{{\rm{4}}{\rm{.9}}\; \times \;{\rm{1}}{{\rm{0}}^{{\rm{ – 10}}}}}}\; = \;{\rm{2}}{\rm{.0 }} \times {\rm{ 1}}{{\rm{0}}^{{\rm{ – 5}}}}\]

 

Step 5. Set up an ICE table for K_b assigning x mol/l for ionization.

 

 

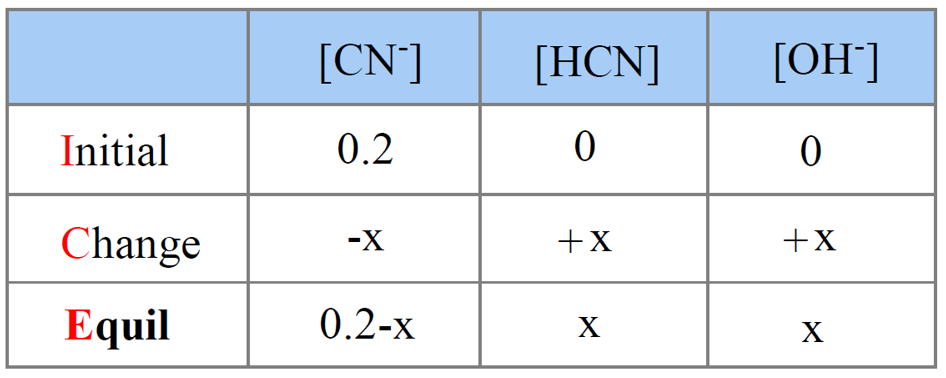
Step 6. Write the expression for K_b using the equilibrium concentration sin the ICE table in order to determine [OH^–].

 

\[{K_{\rm{b}}}\; = \,\frac{{{\rm{[HCN][O}}{{\rm{H}}^{\rm{ – }}}{\rm{]}}}}{{{\rm{[C}}{{\rm{N}}^{\rm{ – }}}{\rm{]}}}}\; = \;\frac{{{{\rm{x}}^{\rm{2}}}}}{{{\rm{0}}{\rm{.20}}\;{\rm{ – }}\;{\rm{x}}}}\; \approx \;\frac{{{{\rm{x}}^{\rm{2}}}}}{{{\rm{0}}{\rm{.20}}\;}}\; = \;2.0\; \times \;{10^{ – 5}}\]

 

Solving for x, we find that x = 0.0020 mol/l and we check if the approximation was valid:

 

\[\% \, = \;\frac{{0.0020}}{{0.20}}\; \times \;100\% \; = \;1.0\% \]

 

The approximation is valid, so the concentration of OH^– ions at equilibrium is 0.0020 M.

 We can now calculate the pOH, and then pH using the pH + pH = 14 relationship:

 

pOH = -log 0.0020 = 2.7, and therefore, the pH is:

pH = 14 – pOH = 14 – 2.7 = 11.3

 

And this answer is also reasonable because we predicted to have a basic solution.

 

Salts the Yield Neutral Solutions

We have seen earlier that a salt with a cation and an anion of a strong base and a strong acid, yields a neutral solution because the ions do not react with water, and thus, no H₃O⁺ or OH^– is generated. For example, the table salt NaCl fount also in physiological solutions has a negligible effect on the pH of the solution.

In the next post, we will discuss determining the pH of a salt prepared from a weak and a weak base.

 

Check Also

• Definitions of Acids and Bases
• Acid-Base Reactions
• Acid-Base Titrations
• Conjugate Acid and Conjugate Base
• Autoionization of Water and K_w
• The pH and Acidity
• Acid Strength, K_a, and pK_a
• Base Strength, K_b and pK_b
• K_a, pK_a, K_b, and pK_b Relationship
• The pH of a Strong Acid and Base
• pH + pOH = 14
• The pH of a Weak Acid
• The pH of a Weak Base
• ThepH of Polyprotic Acids
• The acidity of a Salt Solution
• The pH of a Salt Solution
• The pH of Salts With Acidic Cations and Basic Anions
• Acids and Bases Practice Problems