We saw, in the previous post, how the reaction quotient is used to predict the direction of a reaction before an equilibrium is reached.
To do so, we plug the values of initial concentrations in the expression of the equilibrium constant and, depending on how the values of the quotient and equilibrium constant relate, we predict the direction of the reaction.
Remember, if;
- Q < K Reaction tends to form more products.
- Q > K Reaction tends to form more reactants.
- Q = K Reaction is already at equilibrium.
Also, if any reactant or product is missing in the initial mixture, the reaction will shift in the direction forming some of that component.
So, let’s see how the reaction quotient is used to determine the equilibrium concentrations.
For example, let’s consider the decomposition reaction of POCl3 to POCl and Cl2 gases.
POCl3(g) ⇌ POCl(g) + Cl2(g) Kc = 0.650
If the following amounts of reactants and products were mixed, what will the equilibrium concentration of all components be?
[POCl3] = 0.650 M, [POCl] = 0.450 M, and [Cl2] = 0.250 M
Solution
These are the initial concentrations of the components and, most likely, they are different than what we’ll have at equilibrium. They would be equal to equilibrium concentrations if Q = K and this is what we need to find out.
So, the first step is to determine the reaction quotient and thus the direction of the reaction.
\[Q\;{\rm{ = }}\;\frac{{\left[ {{\rm{POCl}}} \right]\left[ {{\rm{C}}{{\rm{l}}_{\rm{2}}}} \right]}}{{\left[ {{\rm{POC}}{{\rm{l}}_3}} \right]}}\;\]
\[Q\;{\rm{ = }}\;\frac{{{\rm{0}}{\rm{.450 }} \times \;{\rm{0}}{\rm{.250}}}}{{0.650}}\; = 0.173\]
Because Q < K, the reaction will proceed to the right. Remember, the tendency is to reach equilibrium, and therefore, Q “wants to” increase and become equal to the K. Q will increase if more products are formed since their concentrations appear in the numerator.
This means, the concentrations of POCl and Cl2 are going to increase, while the concentration of POCl3 will decrease.
Once we know the direction of the reaction, we are going to set up what is called an ICE table. ICE is an abbreviation for Initial, Change, Equilibrium concentrations of the reactants and products.
This is how we label the rows and columns in the table:
POCl3(g) ⇌ POCl(g) + Cl2(g)
| [POCl3] | [POCl] | [Cl2] | |
| Initial | |||
| Change | |||
| Equil |
We know the initial concentrations ([POCl3] = 0.650 M, [POCl] = 0.450 M, and [Cl2] = 0.250 M) as they are given in the problem. The “change” is the amounts of the components that react before the equilibrium is established.
Since we don’t know this, we assign x mol/L of POCl3 as the reacted amount. Next, we determine how much of each product will be formed if x mol/L of POCl3 decomposes (reacts). This is done based on the stoichiometric ratio of the components, and because it is 1 : 1 mole ratio between all the components, there is going to be x mol/L of POCl(g) and x mol/L Cl2(g) formed.
We can put x on top/bottom of each component in the chemical equation:
x x x
POCl3(g) ⇌ POCl(g) + Cl2(g)
Now, instead of simply using x, we also add a sign to it. In this case, for the reactant(s), it is going to “-x” because its concertation is decreasing, and for the products, it is “+x” since their concertation is increasing:
-x +x +x
POCl3(g) ⇌ POCl(g) + Cl2(g)
At his point, we know the initial concentrations (I in the table), and the change in concentrations (c in the table) which is how much the concentration of the reactant has decreased, and the ones of the products have increased.
So, let’s write these in the table:
POCl3(g) ⇌ POCl(g) + Cl2(g)
| [POCl3] | [POCl] | [Cl2] | |
| Initial | 0.650 | 0.45 | 0.250 |
| Change | -x | +x | +x |
| Equil |
The last part to fill up the table is determining the equilibrium concentrations. And, since we have already set the sign for x, all you need to do here is add the values in the “initial” and “change” cells:
POCl3(g) ⇌ POCl(g) + Cl2(g)
| [POCl3] | [POCl] | [Cl2] | |
| Initial | 0.650 | 0.45 | 0.250 |
| Change | -x | +x | +x |
| Equil | 0.650-x | 0.450+x | 0.250+x |
Now that we have the expressions for equilibrium concentrations, we can plug them in the equation for the equilibrium constant and find the concentrations by solving for the x:
\[K\;{\rm{ = }}\;\frac{{\left[ {{\rm{POCl}}} \right]\left[ {{\rm{C}}{{\rm{l}}_{\rm{2}}}} \right]}}{{\left[ {{\rm{POC}}{{\rm{l}}_3}} \right]}}\;\]
\[K\;{\rm{ = }}\;\frac{{\left( {{\rm{0}}{\rm{.450 + x}}} \right)\left( {{\rm{0}}{\rm{.250 + x}}} \right)}}{{0.650 – x}}\; = 0.650\]
We have a quadratic equation which is what you are going to work with for most problems on equilibrium concentrations. First, we simplify it to be able to use the formula for quadratic equations:
0.1125 + 0.450x + 0.250x + x2 = 0.4225 – 0.650x
0.1125 + 0.700x + x2 = 0.4225 – 0.650x
x2 + 1.35x – 0.310 = 0
\[{\rm{x}}\;{\rm{ = }}\;\frac{{ – b \pm \sqrt {{b^2} – 4ac} }}{{2a}}\]
a = 1, b = 1.35, c = -0.310
Therefore,
\[{\rm{x}}\;{\rm{ = }}\;\frac{{ – 1.35\, \pm \,\sqrt {1.8225\; – \,4\; \cdot \;1\; \cdot \;( – 0.310)} }}{{2 \cdot \;1}}\]
x = 0.200 or x= -1.55
x=-1.55 doesn’t work because that would indicate increasing the concentration of POCl3, and therefore,
x=0.200
Now, this is how much POCl3 has reacted, and to find the equilibrium concatenations, we go based on the expressions in the ICE table:
[POCl3] at equilibrium is 0.650 – 0.200 = 0.450 mol/L
[POCl] at equilibrium is 0.450 + 0.200 = 0.650 mol/L
[Cl2] at equilibrium is 0.250 + 0.200 = 0.450 mol/L
It is always a good idea to plug this numbers in the expression for Kc and check if they are correct:
\[K\;{\rm{ = }}\;\frac{{\left[ {{\rm{POCl}}} \right]\left[ {{\rm{C}}{{\rm{l}}_{\rm{2}}}} \right]}}{{\left[ {{\rm{POC}}{{\rm{l}}_3}} \right]}}\; = \;\frac{{(0.650)(0.450)}}{{(0.450)}}\; = \;0.650\;\;\]
![]()
Can We Do It Without Solving Quadratic Equations?
The good news is that yes, most often you can determine the equilibrium concentrations without solving a quadratic equation.
This is possible for reactions with a small equilibrium constant (in the 10-3 and below range).
Let’s see how it works in the following example.
Consider the following equilibrium:
2NOCl(g) ⇆ 2NO(g) + Cl2(g)
2.00 mole of pure NOCl and 1.65 mole of pure Cl2 are placed in a 1.00-L container. Calculate the equilibrium concentration of NO(g) considering that with K = 2.4 x 10–6.
Again, the first thing here is to determine the direction of the reaction. There are both reactants and products in the initial mixture, however, there is no NO, and remember, when one of the reactants or products is missing from the system, the equilibrium is going to be established by producing some of that compound. Therefore, the reaction is going to proceed to the right to produce some NO and we don’t need to (we cannot actually) calculate the reaction quotient.
So, let’s set up an ICE table and, for convivence, assign 2x as the depletion in NOCl concentration:
2NOCl(g) ⇆ 2NO(g) + Cl2(g)
| [NOCl] | [NO] | [Cl2] | |
| Initial | 2.00 | 0 | 1.65 |
| Change | -2x | +2x | +x |
| Equil | 2.00-2x | 2x | 1.65+x |
The equilibrium constant is:
\[K = \frac{{{{[{\rm{NO}}]}^{\rm{2}}}[{\rm{C}}{{\rm{l}}_{\rm{2}}}]}}{{{{[{\rm{NOCl}}]}^{\rm{2}}}}}\]
\[K = \;\frac{{{{\left( {{\rm{2x}}} \right)}^{\rm{2}}}\left( {{\rm{1}}{\rm{.65 + x}}} \right)}}{{{{\left( {{\rm{2}}{\rm{.00 – 2x}}} \right)}^{\rm{2}}}}}\;{\rm{ = }}\;{\rm{2}}{\rm{.4 \times 1}}{{\rm{0}}^{{\rm{–6}}}}\]
This is a cubic equation, and what you can do it these situations, is make an approximation that 2x<<2.00 or x<<1.00 because the equilibrium constant a very small and the amount of the reactant that reacts is going to be insignificant compared to its initial quantity.
This allows to simplify the equation as follows:
\[K = \;\frac{{{{\left( {{\rm{2x}}} \right)}^{\rm{2}}}\left( {{\rm{1}}{\rm{.65}}} \right)}}{{{{\left( {{\rm{2}}{\rm{.00}}} \right)}^{\rm{2}}}}}\;{\rm{ = }}\;{\rm{2}}{\rm{.4 \times 1}}{{\rm{0}}^{{\rm{–6}}}}\]
\[K = \;\frac{{{\rm{4}}{{\rm{x}}^{\rm{2}}}\left( {{\rm{1}}{\rm{.65}}} \right)}}{{\rm{4}}}\;{\rm{ = }}\;{\rm{2}}{\rm{.4 \times 1}}{{\rm{0}}^{{\rm{–6}}}}\]
6.6x2 = 9.6×10-6
x = 0.00121
The equilibrium concentration of NO is 2x, so that is 0.00242 mol/L.
Now, we need to plug the numbers and see if the value for the equilibrium constant is close enough to the given number (K = 2.4 x 10–6).
\[K = \;\frac{{{{\left( {{\rm{2x}}} \right)}^{\rm{2}}}\left( {{\rm{1}}{\rm{.65 + x}}} \right)}}{{{{\left( {{\rm{2}}{\rm{.00 – 2x}}} \right)}^{\rm{2}}}}}\;{\rm{ = }}\;{\rm{2}}{\rm{.4 \times 1}}{{\rm{0}}^{{\rm{–6}}}}\]
\[K = \;\frac{{{{\left( {{\rm{2}} \times {\rm{0}}{\rm{.00121}}} \right)}^{\rm{2}}}\left( {{\rm{1}}{\rm{.65 + 0}}{\rm{.00121}}} \right)}}{{{{\left( {{\rm{2}}{\rm{.00 – 2}} \times {\rm{0}}{\rm{.00121}}} \right)}^{\rm{2}}}}}\;{\rm{ = }}\;{\rm{2}}{\rm{.42 \times 1}}{{\rm{0}}^{{\rm{–6}}}}\]
And that is very close to the value of K, and therefore, out approximation was correct, and the equilibrium concentration of NO is 0.00242 mol/L.
Usually, the approximation is considered to be valid if the x is less than 5% of the reactant’s initial
concentration (less than 5% of it reacts).
So, to find this percentage, we divide the reactant amount over the initial concentration of NOCl:
0.00242/2.00 x 100% = 0.121%
Therefore, the approximation was valid. If you determine the parentage and find out that it was not, the equation must be solved without the shortcut.
In the end, let’s enter the values for the equilibrium concentrations to make sure everything is correct:
\[K\; = \;\frac{{{{\left( {{\rm{2}}\, \times \;{\rm{0}}{\rm{.00121}}} \right)}^{\rm{2}}}\left( {{\rm{1}}.{\rm{65}}\,{\rm{ + }}\;{\rm{0}}{\rm{.00121}}} \right)}}{{{{\left( {{\rm{2}}.{\rm{00}}\;{\rm{ – }}\;{\rm{2}}\, \times \;{\rm{0}}{\rm{.00121}}} \right)}^{\rm{2}}}}}\;{\rm{ = }}\;{\rm{2}}.{\rm{4\;}} \times \;{\rm{1}}{{\rm{0}}^{{\rm{ – 6}}}}\]
![]()
Practice
The equilibrium constant for the following reaction at 600 oC is determined to be Kc = 0.495:
H2O(g) + CO(g) ⇆ H2(g) + CO2(g)
Calculate the number of H2 moles that are present at equilibrium if a mixture of 0.400 mole CO and 0.500 mole H2O is heated to 600°C in a 10.0-L container.
The equilibrium constant Kc for the following reaction at 800°C is 3.74 x 105
H2(g) + I2(g) ⇆ 2HI(g)
If 6.25 moles of HI were initially added to a 15.0-L empty vessel, what would the concentrations of H2, I2, and HI be at equilibrium.
The equilibrium constant for the following reaction at 700 K is Kp = 6.7 x 10-3
CO(g) + 2H2(g) ⇆ CH3OH(g)
A reaction mixture contains 0.248 atm of H2, 0.085 atm of CO, and 0.598 atm of CH3OH. Is the reaction mixture at equilibrium? If not, in what direction will the reaction proceed?
For the reaction shown below, Kc = 0.654 at 600 K.
N2O4(g) ⇆ 2NO2(g)
If initially, 0.0600 M of N2O4 are present in the reaction vessel, what are the equilibrium concentrations of the gases at 600 K?
For the reaction shown below, Kc = 255 at 800 K.
PCl3(g) + Cl2(g) ⇆ PCl5(g)
If a reaction mixture initially contains 0.3500 M PCl3 and 0.375 M Cl2 at 800 K, what are the equilibrium concentrations of all the species in the mixture?
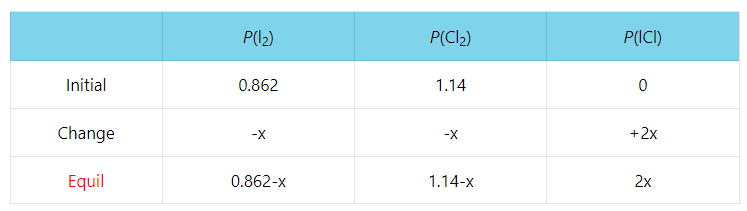
Consider the following reaction characterized with Kp = 2.34 x 10-4 at 250 K:
I2(g) + Cl2(g) ⇆ 2ICl(g)
A reaction mixture initially contains I2 with partial pressure of 655 torr and Cl2 with partial pressure of 864 torr at 250 K. Calculate the equilibrium partial pressure of ICl.
Consider the following equilibrium:
2NO(g) + 2H2(g) ⇆ N2(g) + 2H2O(g)
Initially, there are 0.15 moles of NO and 0.25 moles of H2, in a 10.0-L container. If there are 0.056 moles of NO at equilibrium, how many moles of N2 are present at equilibrium?
Check Also
- Chemical Equilibrium
- Equilibrium Constant
- Kpand Partial Pressure
- Kp and KcRelationship
- K Changes with Chemical Equation
- Equilibrium Constant K from Two Reactions
- Reaction Quotient – Q
- ICE Table – Calculating Equilibrium Concentrations
- ICE Table Practice Problems
- Le Châtelier’s principle
- Le Châtelier’s principle Practice Problems
- Chemical Equilibrium Practice Problems